Chapter 2 Limits And Derivatives 2.1 Exercises
A A b B. 542 Evaluate a triple integral by expressing it as an iterated integral.

Limits Introduction Properties And Algebra Of Limits Videos Examples
211 Describe the tangent problem and how it led to the idea of a derivative.

. 352 Find the derivatives of the standard trigonometric functions. Enter the email address you signed up with and well email you a reset link. Introduction to Systems of Equations and Inequalities.
543 Recognize when a function of three variables is integrable over a closed and bounded region. Just as when we found the derivatives of other functions we can find the derivatives of exponential and logarithmic functions using formulas. π 2 π 2.
93 Systems of Nonlinear Equations and Inequalities. Republiken Finland listen to all is a Nordic country in Northern Europe. Introduction to Systems of Equations and Inequalities.
233 Evaluate the limit of a function by. Fx -3x2 12x5 fx_____. We now turn our attention to finding derivatives of inverse trigonometric functions.
212 Explain how the idea of a limit is involved in solving the tangent problem. 541 Recognize when a function of three variables is integrable over a rectangular box. Figure 326 These graphs show two important limits needed to establish the derivative formulas for.
Derivatives of Inverse Trigonometric Functions. 92 CHAPTER 2 Limits and Derivatives 22. 321 Define the derivative function of a given function.
95 Matrices and Matrix Operations. Introduction to Systems of Equations and Inequalities. Helsinki is the capital and largest city forming a larger metropolitan area with the neighbouring cities of Espoo Kauniainen and VantaaThe vast majority of the population.
91 Systems of Linear Equations. 97 Solving Systems with Inverses. 96 Solving Systems with Gaussian Elimination.
213 Determine the area of a region between two curves by integrating with respect to the dependent variable. 95 Matrices and Matrix Operations. A As x the values of f are getting arbitrarily close to LThe line yL is a horizontal asymptote of f.
For example consider a simple tax system in which incomes up to 10000 are taxed at 10 and any additional income is taxed at 20. Quotation and trade reporting system. 23 The Limit Laws.
96 Solving Systems with Gaussian Elimination. 21 A Preview of Calculus. 412 Sketch a graph of a function of two variables.
215 Describe the area problem. 231 Recognize the basic limit laws. 431 Calculate the partial derivatives of a function of two variables.
This chapter briefly summarizes the shells building. Tax brackets are another real-world example of piecewise functions. 92 Systems of Linear Equations.
325 Explain the meaning of a higher-order derivative. 411 Recognize a function of two variables and identify its domain and range. 92 Systems of Linear Equations.
22 The Limit of a Function. 93 Systems of Nonlinear Equations and Inequalities. 322 Graph a derivative function from the graph of a given function.
Bash is an acronym for Bourne-Again SHellThe Bourne shell is the traditional Unix shell originally written by Stephen Bourne. Describe all numbers x x that are at a distance of 1 2 1 2 from the number 4. Explore Features The Right Content at the Right Time Enable deeper learning with expertly designed well researched and time-tested content.
All of the Bourne shell builtin commands are available in Bash The rules for evaluation and quoting are taken from the POSIX specification for the standard Unix shell. 232 Use the limit laws to evaluate the limit of a function. 433 Determine the higher-order derivatives of a function of two variables.
Microsoft pleaded for its deal on the day of the Phase 2 decision last month but now the gloves are well and truly off. At the same time it may seem odd to use a linear approximation when we can just push a. 93 Systems of Nonlinear Equations and Inequalities.
The value given by the linear approximation 30167 is very close to the value obtained with a calculator so it appears that using this linear approximation is a good way to estimate x x at least for x x near 9. 432 Calculate the partial derivatives of a function of more than two variables. Fx2x1 at x 5Use the short cut theorems to take and evaluate the following derivativesSimplify the answer as much as possible.
324 Describe three conditions for when a function does not have a derivative. 212 Find the area of a compound region. 214 Identify instantaneous velocity as the limit of average velocity over a small time interval.
414 Recognize a function of three or more variables and identify its level surfaces. This equation is correct if x x belongs to the restricted domain π 2 π 2 π 2 π 2 but sine is defined for all real input values and for x x outside the restricted interval the equation is not correct because its inverse always returns a value in π 2 π 2. 213 Recognize a tangent to a curve at a point as the limit of secant lines.
2121 1 The Commission may on the application of a quotation and trade reporting system recognize the quotation and trade reporting system if the Commission is satisfied that to do so is in the public interest. Learn More Improved Access through Affordability Support student success by choosing from an array of. 96 Solving Systems with Gaussian Elimination.
Finland ˈfɪnland officially the Republic of Finland Finnish. These derivatives will prove invaluable in the study of integration later in this text. Find the equation of the tangent line to the graph of x 3 x ln y y 3 2 x 5 x 3 x.
434 Explain the meaning of a partial differential equation and give an example. 211 Determine the area of a region between two curves by integrating with respect to the independent variable. Microsoft describes the CMAs concerns as misplaced and says that.
3 Basic Shell Features. 353 Calculate the higher-order derivatives. 92 Systems of Linear Equations.
413 Sketch several traces or level curves of a function of two variables. 323 State the connection between derivatives and continuity. Cartesian product A B of two sets A and B is given by A B a b.
91 Systems of Linear Equations. 91 Systems of Linear Equations. 97 Solving Systems with Inverses.
95 Matrices and Matrix Operations. 97 Solving Systems with Inverses. The tax on a total income S S would be 01 S 01 S if S 10 000 S 10 000 and 1000 02 S 10 000 1000 02 S.
Miscellaneous Exercise on Chapter 2 Solutions 12 Questions. The two elements which are grouped in a particular order are termed an ordered pair. 2 1 2 - 01032011.
544 Simplify a calculation by changing the order of integration of a triple integral. The situation is similar for cosine and. Using the limit definition of a derivative find the derivative at a point for.
351 Find the derivatives of the sine and cosine function. Key Features of NCERT Solutions for Class 11 Maths Chapter 2 Relations and Functions. Using a calculator the value of 91 91 to four decimal places is 30166.
For the following exercises solve the equations below and express the answer using set. The derivatives of inverse trigonometric functions are quite surprising in that their derivatives are actually algebraic. Flexibility at Every Step Build student confidence problem-solving and critical-thinking skills by customizing the learning experience.
Express this using absolute value notation.

Solution Manual Chapter 02 Limits And Continuity

Ncert Solutions For Class 11 Maths Chapter 13 Limits And Derivatives Merit Batch

Unit 02 Differentiation Mathcity Org
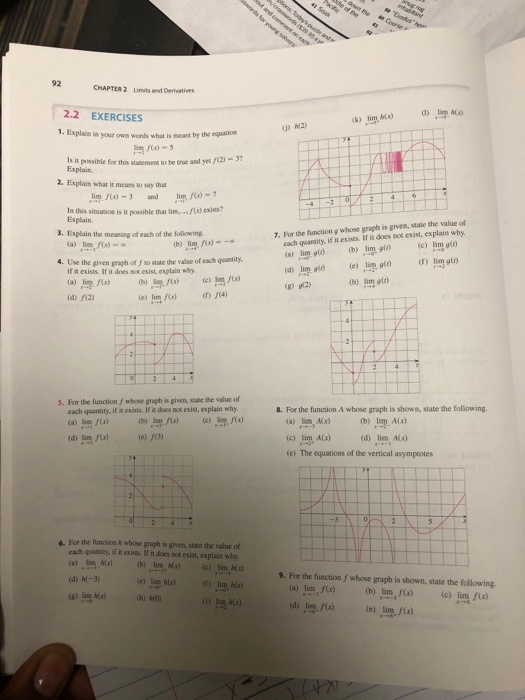
Solved 92 Chapter 2 Limits And Derivatives 2 2 Exercises M2 Chegg Com

Limits And Derivatives Exercise 13 2 Ncert Solutions

Calculus Of A Single Variable 8th Edition Chapter 2 Answer Key Pdf Derivative Slope
Chapter 3 Derivatives Mathgotserved

Ncert Solutions For Class 11 Maths Chapter 13 Limits And Derivatives Class 11 Maths

Ncert Solutions For Class 11 Maths Chapter 2 Exercise 2 1 Embibe
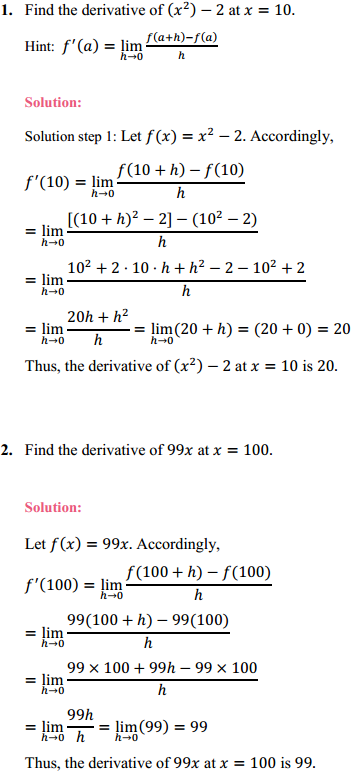
Ncert Solutions For Class 11 Maths Chapter 13 Limits And Derivatives Ex 13 2

Ncert Solutions For Class 11 Maths Chapter 2 Relations And Functions Free Pdf Download

Ncert Solutions For Class 11 Maths Chapter 2 Relations And Functions Free Pdf Download

Limits And Derivatives Exercise 13 1 Ncert Solutions
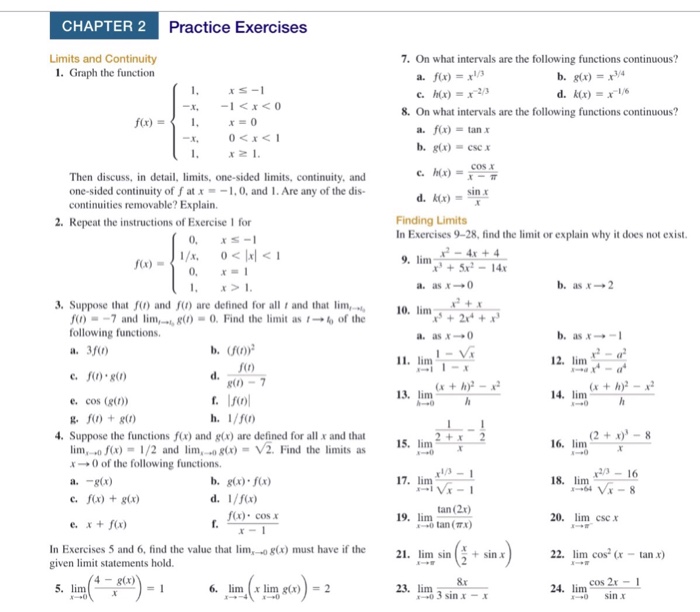
Chapter 2 Practice Exercises Limits And Continuity 1 Chegg Com
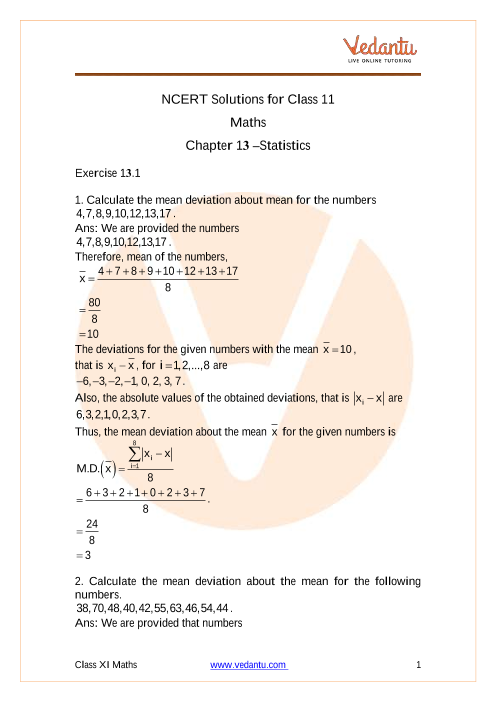
Ncert Solutions For Class 11 Maths Exercise 13 1 Chapter 13 Limits And Derivatives
Solution Manual Chapter 02 Limits And Continuity
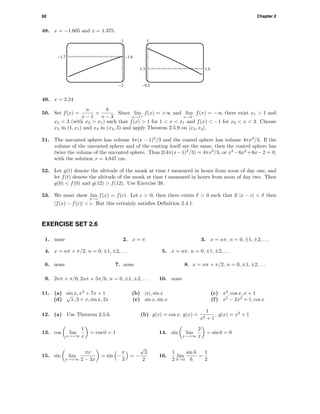
Solution Manual Chapter 02 Limits And Continuity